Solving Algebra Equations with Variables on Both Sides

Solving algebra equations with variables on both sides is really tough. But, you will have no problems solving these equations after you follow the step-by-step instructions in these 3 examples.

So far, you've learned how to solve one-step equations, two-step equations, and equations with fractions. If you haven't mastered these skills or need a refresher, please go back and review these lessons before attempting this one! It will be so much easier if you have the background!

So, if you are ready, let's move on!



Solving algebra equations with variables on both sides look intimidating, but they really aren't that hard if you have the background skills. In fact, I think these types of equations are fun because it's like moving pieces of a puzzle around.

This is what you need to remember:


Solving Equations With Variables on Both Sides

  • If you have any fractions, get rid of those first by multiplying ALL terms by the denominator.
  • Use the distributive property if needed.
  • Your ultimate goal is to get all of the constants on one side of the equation and all of the variables on the other side of the equation. You can accomplish this by adding or subtracting terms on BOTH sides of the equation.

Let's take a look at a few examples.


Example 1 - Variables on Both Sides


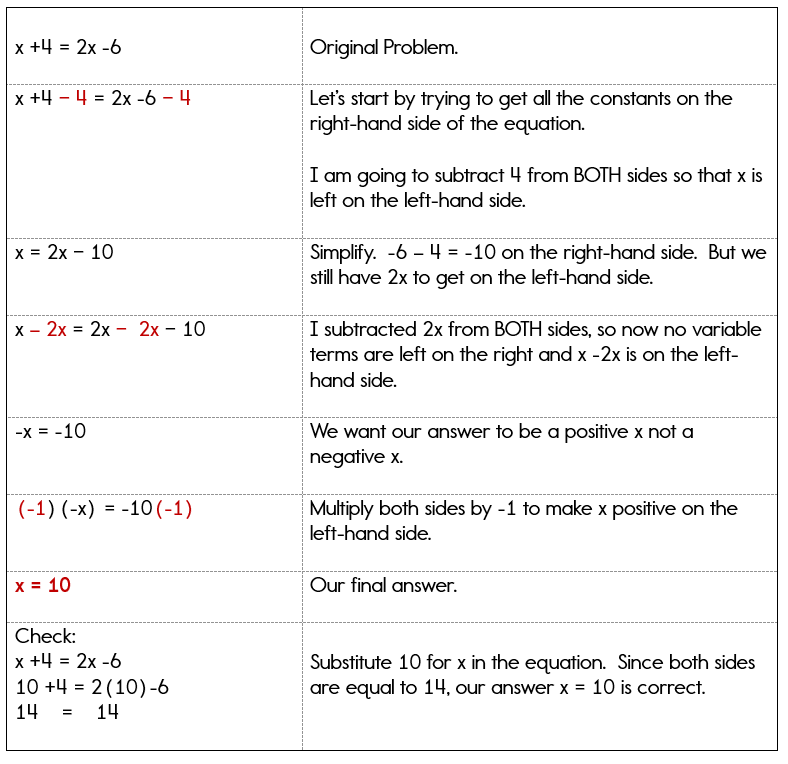
Did you notice how we used all of the rules we learned in previous lessons?

Remember, our goal is to get all of the variables on one side and all the constants on the other side.

This next example shows how to solve if the distributive property is involved.


Example 2 - Working with the Distributive Property


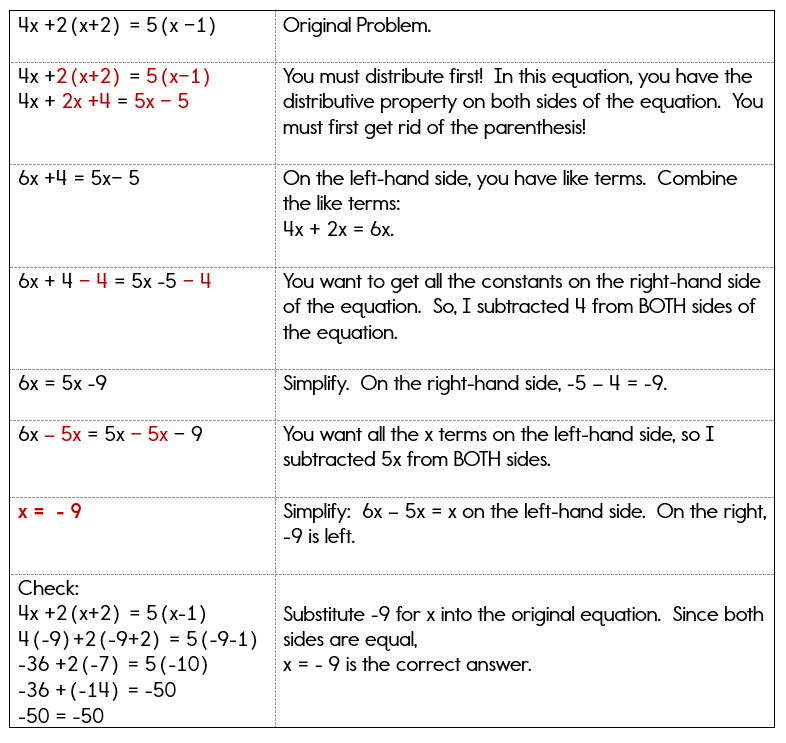
Yes, this is a confusing topic, so if you are still having trouble, check out example 2 on video.




Now let's see what happens when we bring it all together and try to solve an equation that has fractions and variables on both sides!


Example 3 - Fractions and Variables on Both Sides


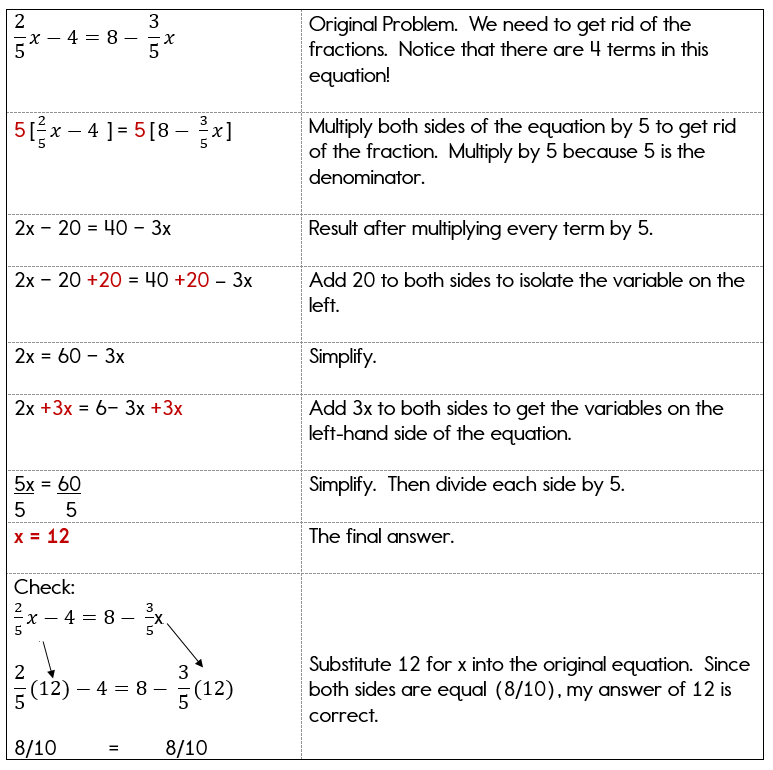
It doesn't look too hard, does it? Remember: it's like moving puzzle pieces around - all the variable terms on one side and all the constant terms on the other side!





  1. Home
    2. >
  2. Solving Equations
    3. >
  3. Balancing Equations



Comments

We would love to hear what you have to say about this page!

Need More Help With Your Algebra Studies?

Get access to hundreds of video examples and practice problems with your subscription! 

Click here for more information on our affordable subscription options.

Not ready to subscribe?  Register for our FREE Pre-Algebra Refresher course.