Solving One-Step Equations
Multiplication Equations
The next set of one-step equations do not contain a constant that you must add or subtract to remove.
These equations contain a coefficient. A coefficient is a number that is multiplied by the variable.
Therefore, we must remove the coefficient. Take a look at this equation: 3x = 9. Since there is no mathematical symbol between the 3 and the x we know that means multiplication. So, what number times 3 will give us an answer of 9?
You know the answer, right? Yes, 3! 3*3 = 9
Another question to ponder...
What is the opposite of multiplication? Yes... division!
We are going to divide in order to get x by itself!
Why divide? What is 3/3? Yes... 1! What is 1*x? You got it.... x! That's how we get x by itself.
We want the coefficient to be 1. Anytime you divide a number by itself, you will get an answer of 1!
Let's look at a few examples:
Example 1- One-step Multiplication Equations
Pretty easy, huh? I think multiplication equations are even easier than addition and subtraction equations. Keep working, you'll get the hang of it!
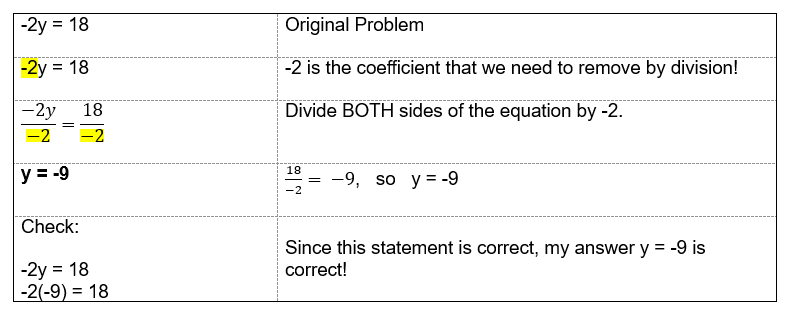
Example 2 - Working with Negative Numbers
Not too hard if the coefficient is an integer! What happens if the coefficient is a fraction?
What Happens When the Coefficient is a Fraction?
Think:
If I have 2/3x, how can I make 2/3 a coefficient of 1?
Yes... you will divide by 2/3, but...Do you remember the term reciprocal?
When you divide a fraction, you actually multiply by the reciprocal. If you take 2/3 and you flip it to 3/2, that is the reciprocal!
If you multiply by the reciprocal, you will have a coefficient of 1.
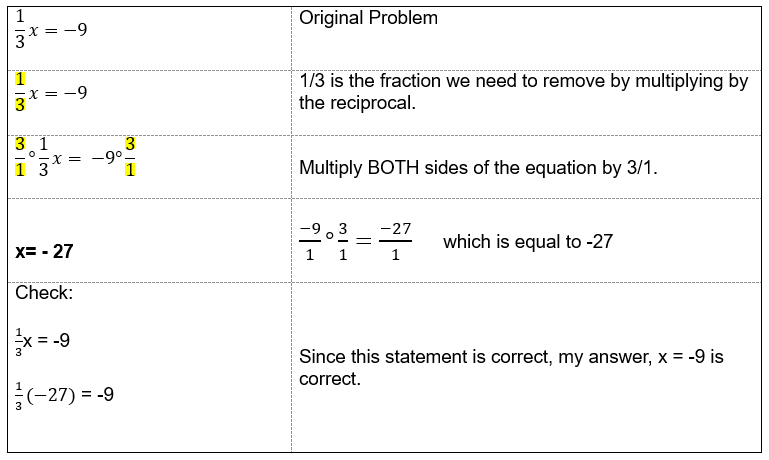
Example 3: Multiplication Equations with a Fraction Coefficient
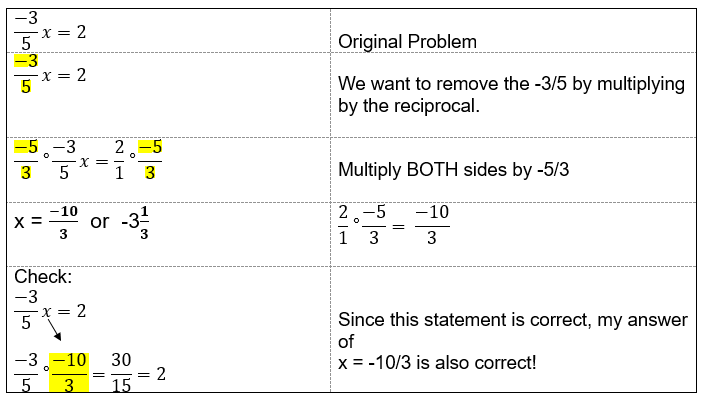
You may also have problems where your answer results in a fraction. Here's one more example:
Example 4: Multiplication Equations
In this lesson, you learned a new vocabulary word, "coefficient" and hopefully you now have a better understanding of how to solve multiplication equations.
Need More Help With Your Algebra Studies?
Get access to hundreds of video examples and practice problems with your subscription!
Click here for more information on our affordable subscription options.
Not ready to subscribe? Register for our FREE Pre-Algebra Refresher course.
Need Help? Try This Online Calculator!
Affiliate Products...
On this site, I recommend only one product that I use and love and that is Mathway If you make a purchase on this site, I may receive a small commission at no cost to you.
Comments
We would love to hear what you have to say about this page!