Using the Substitution Method to
Solve Systems of Equations
The substitution method is one of two ways to solve systems of equations without graphing.
You might ask yourself, "Why wouldn't I just want to graph the equations to find the solution?" Well there are many reasons...
You may not have graph paper or an accurate way to graph the equations, thus making it hard to identify the solution.
Or, as the equations become more difficult, the solution is not always an identifiable point on the graph. This means that the solution may contain decimals or fractions, which is not easy to identify on a graph.
Once you learn the algebraic method for solving a system of equations, you will probably find that it becomes your preferred method. Graphing becomes too tedious.
The good news is that there are two methods, which makes this process easier depending on the problems you are given.
So, let's get to it!
The following steps can be used as a guide as you read through the examples for using the substitution method.
Steps for Using the Substitution Method in order to Solve Systems of Equations
- Solve 1 equation for 1 variable. (Put in y = or x = form)
- Substitute this expression into the other equation and solve for the missing variable.
- Substitute your answer into the first equation and solve.
- Check the solution.
These directions will make a lot more sense when you study the examples below.
Example 1 - Using Substitution to Solve a System of Equations
Solve the following system of equations by using substitution.
-x + y = 1
2x + y = -2
Solution
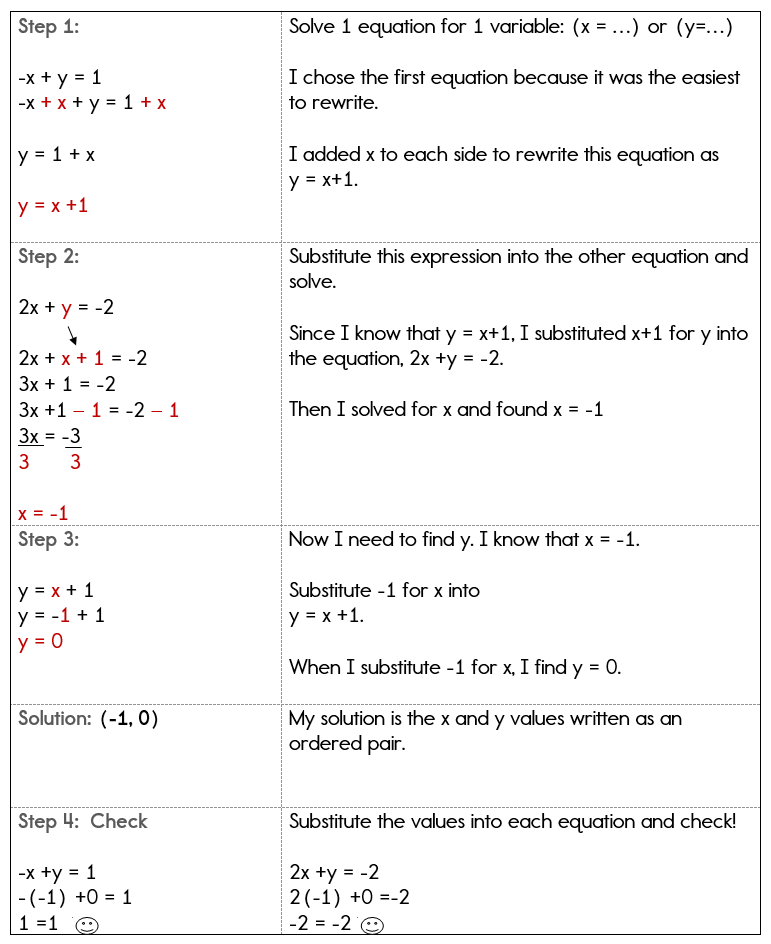
The next example demonstrates a situation where it is easier to solve for x initially.
Example 2: Solving a System of Equations
Solve the following system of equations by using substitution.
2x + 2y = 3
x - 4y = -1
Solution
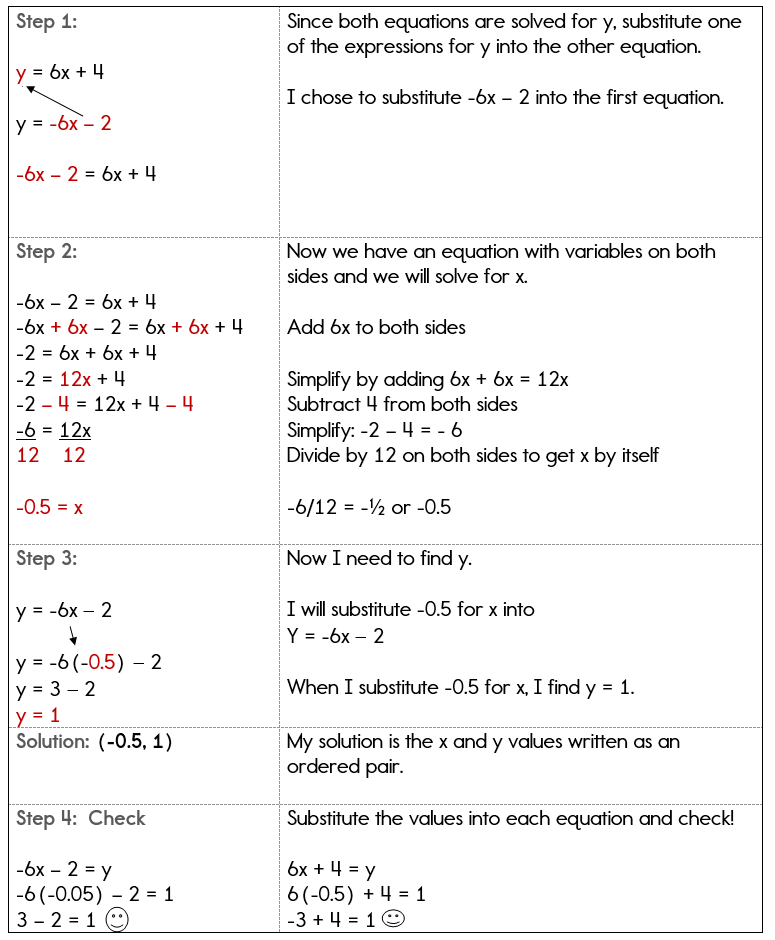
We have another example where the original system of equations is easily solved by using substitution. In this case, both equations are already solved for a variable; therefore, we can substitute one expression for y and solve!
Take note of how we have an equation with variables on both sides.
Example 3: Using Substitution to Solve a System of Equations
Use substitution to solve the following system of equations:
y = 6x + 4
y = -6x - 2
Solution
Let's take a look at another example where you will find that you cannot solve the system. What happens when you can't solve?
You will have no solution!
Pay close attention to the very last step of the solution.
Example 4: No Solution
Solve the following system of equations.
3x - y = 2
6x - 2y = -8
Solution
We have a problem! 6x- 6x = 0. Since my x terms cancel out, we are left with 4 = -8.
This is NOT a true statement.
Therefore, this is not a solution. That means that there is NO SOLUTION to this system of equations.
Can you imagine what type of graph this system represents?
Yes! The lines are parallel. They will never intersect; therefore, there are no solutions.
This is an example of what will happen if you are using the substitution method and there are no solutions. Your end result will not make sense.
Now, let's see what happens if we have an infinite number of solutions. What will our solution look like algebraically?
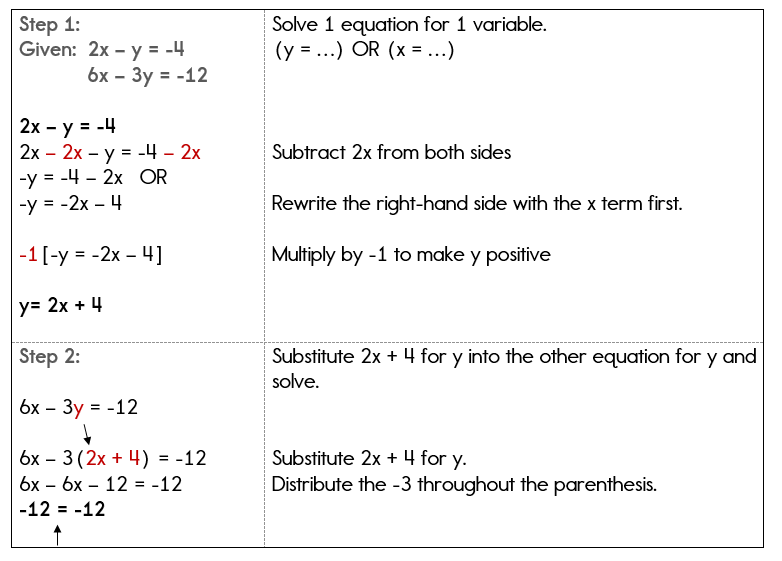
Example 5: An Infinite Number of Solutions
Solve the following system of equations:
2x - y = -4
6x - 3y = -12
Solution
This also looks strange because again, 6x - 6x = 0. They cancel out. We are left with -12 = -12. However, this is a TRUE statement.
Since this is a true statement, there are solutions and this happens to be an infinite number of solutions. Every point on the line is a solution to the system.
What type of graph will this system produce?
You will see two lines lying on top of each other. These are the exact same line and that's why it's an infinite number of solutions.
So, if you are solving a system algebraically and your variables cancel, you will need to see if your end statement makes sense. If it makes sense, you have an infinite number of solutions and if it doesn't make sense, then you have no solution.
Need More Help With Your Algebra Studies?
Get access to hundreds of video examples and practice problems with your subscription!
Click here for more information on our affordable subscription options.
Not ready to subscribe? Register for our FREE Pre-Algebra Refresher course.
Need Help? Try This Online Calculator!
Affiliate Products...
On this site, I recommend only one product that I use and love and that is Mathway If you make a purchase on this site, I may receive a small commission at no cost to you.
Comments
We would love to hear what you have to say about this page!