Greatest Common Factor

Now that you've studied common factors, we are going to take a look at greatest common factors.

Let's take another look at our example of common factors.


Common Factors


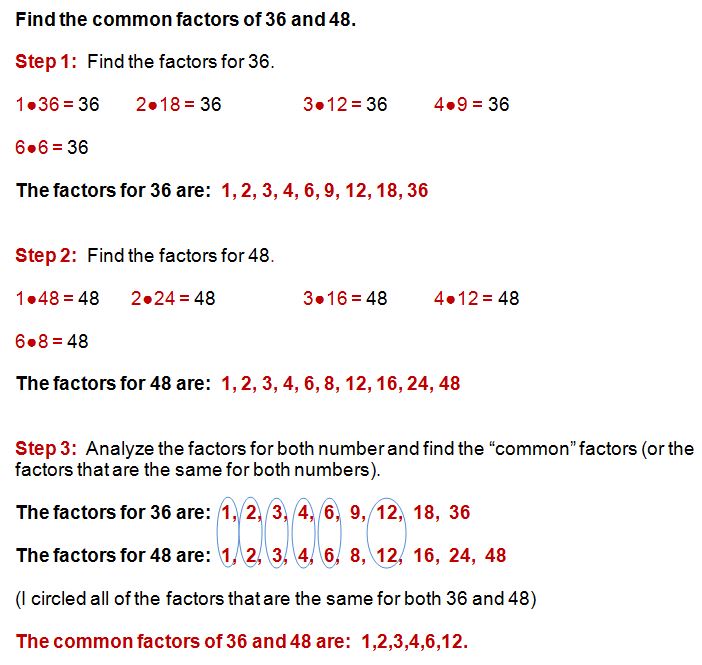
We identified the common factors by circling the factors that were the same for 36 and 48. What if I asked you to identify the Greatest Common Factor?

Yes! That just means, of the common factors, which one is the greatest? For 36 and 48, the Greatest Common Factor is 12. That's the largest number that we circled!


Tip

The Greatest Common Factor is often abbreviated in math, and is known as the GCF.


Let's take a look at another example where you will be asked to find the GCF for three different numbers.


Yes, it does seem like a lot of work, but as you become more comfortable with factors, you may not have to write down all of the factors.

You may be able to mentally identify the GCF for the set of numbers that you are working with.

There are times when you are working with larger numbers and even finding the factors is difficult.

In this case, you may use a technique called prime factorization (or factor trees) to help you to determine the greatest common factor.



  1. Home
    2. >
  2. Fractions
    3. >
  3. GCF



Comments

We would love to hear what you have to say about this page!

Need More Help With Your Algebra Studies?

Get access to hundreds of video examples and practice problems with your subscription! 

Click here for more information on our affordable subscription options.

Not ready to subscribe?  Register for our FREE Pre-Algebra Refresher course.